One of the primary functions of a pavement is load distribution. Therefore, in order to adequately design a pavement something must be known about the expected loads it will encounter. Loads, the vehicle forces exerted on the pavement (e.g., by trucks, heavy machinery, airplanes), can be characterized by the following parameters:
- Tire loads
- Axle and tire configurations
- Repetition of loads
- Distribution of traffic across the pavement
- Vehicle speed
Loads, along with the environment, damage pavement over time. The simplest pavement structural model asserts that each individual load inflicts a certain amount of unrecoverable damage. This damage is cumulative over the life of the pavement and when it reaches some maximum value the pavement is considered to have reached the end of its useful service life.
Therefore, pavement structural design requires a quantification of all expected loads a pavement will encounter over its design life. This quantification is usually done in one of two ways:
- Equivalent single axle loads (ESALs). This approach converts wheel loads of various magnitudes and repetitions (“mixed traffic”) to an equivalent number of “standard” or “equivalent” loads.
- Load spectra. This approach characterizes loads directly by number of axles, configuration and weight. It does not involve conversion to equivalent values. Structural design calculations using load spectra are generally more complex than those using ESALs.
Both approaches use the same type and quality of data but the load spectra approach has the potential to be more accurate in its load characterization.
Tire Loads
Tire loads are the fundamental loads at the actual tire-pavement contact points. For most pavement analyses, it is assumed that the tire load is uniformly applied over a circular area. Also, it is generally assumed that tire inflation and contact pressures are the same (this is not exactly true, but adequate for approximations). The equation below relates the radius of tire contact to tire inflation pressure and the total tire load:
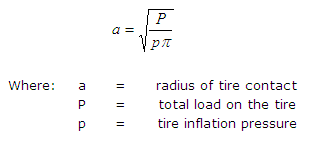
States generally limit the allowable load per inch width of tire. Based on a slightly dated survey (Sharma, Hallin and Mahoney, 1983[1]), this tire load limitation varies from a high of 140 N/mm (800 lbs/inch) to a low of 79 N/mm (450 lbs/inch).

Axle and Tire Configurations
While the tire contact pressure and area is of vital concern in pavement performance, the number of contact points per vehicle and their spacing is also critical. As tire loads get closer together their influence areas on the pavement begin to overlap, at which point the design characteristic of concern is no longer the single isolated tire load but rather the combined effect of all the interacting tire loads. Therefore, axle and tire arrangements are quite important.
Descriptions
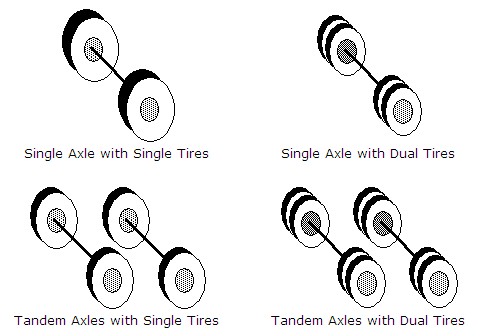
Tire-axle combinations are typically described as (Figure 4):
- Single axle single tire(truck steering axles, etc.)
- Single axle dual tires
- Tandem axle single tires (Figure 5)
- Tandem axle dual tires

Typical Axle Load Limits
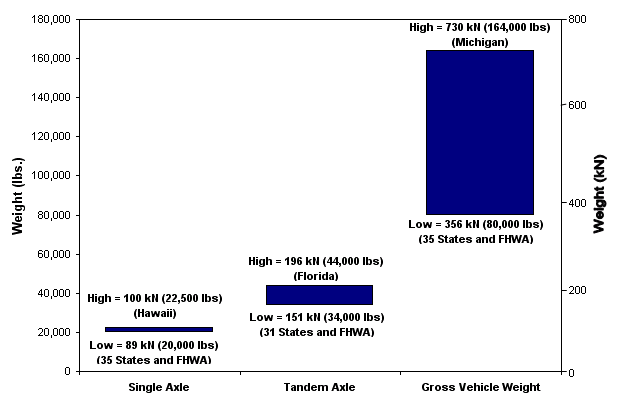
Federal and State laws establish maximum axle and gross vehicle weights to limit pavement damage. The range of weight limits in the U.S. vary a bit based on various Federal and State laws. Figure 6 shows the range of maximum limits for single axle, tandem axle and gross vehicle weight (GVW) established by the states and the FHWA.
Although each state and the FHWA have established maximum axle-tire load combinations, there are other restrictions as well. One of the most common is the FHWA bridge formula (sometimes called the Federal Bridge Formula B).
Repetition of Wheel Loads
Although it is not too difficult to determine the wheel and axle loads for an individual vehicle, it becomes quite complicated to determine the number and types of wheel/axle loads that a particular pavement will be subject to over its entire design life. Furthermore, it is not the wheel load but rather the damage to the pavement caused by the wheel load that is of primary concern.There are currently two basic methods for characterizing wheel load repetitions:
- Equivalent single axle load (ESAL). Based on AASHO Road Test results, the most common approach is to convert wheel loads of various magnitudes and repetitions (“mixed traffic”) to an equivalent number of “standard” or “equivalent” loads. The most commonly used equivalent load in the U.S. is the 80 kN (18,000 lbs) equivalent single axle load (normally designated ESAL).
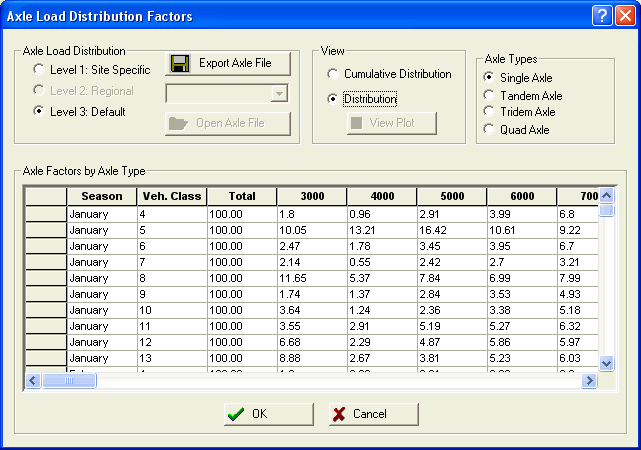
- Load spectra.The 2002 Guide for the Design of New and Rehabilitated Pavement Structures (NCHRP 1-37A) essentially does away with the ESAL and determines loading directly from axle configurations and weights. This is a more precise characterization of traffic but relies on the same input data used to calculate ESALs. typical load spectrum input would be in the form of a table that shows the relative axle weight frequencies for each common axle combination (e.g. single axle, tandem axle, tridem axle, quad axle) over a given time period (Figure 7). Often, load spectra data can be obtained from weigh-in-motion stations.
Typically, designers must not only calculate ESALs or load spectra for various vehicles but also must forecast the expected number of ESALs or load spectra a pavement will encounter over its entire design life. This information then helps determine the structural design. Highway design in most states is based on the ESAL traffic input anticipated over a future 10 to 50 year period.
Traffic Distribution
Along with load type and repetitions, the load distributions across a particular pavement must be estimated. For instance, on a 6-lane interstate highway (3 lanes in each direction) the total number of loads is probably not distributed exactly equally in both directions. Often one direction carries more loads than the other. Furthermore, within that one direction, not all lanes carry the same loading. Typically, the outer most lane carries the most trucks and therefore is subjected to the heaviest loading. Therefore, pavement structural design should account for these types of unequal load distribution. Typically, this is accounted for by selecting a “design lane” for a particular pavement. The loads expected in the design lane are either (1) directly counted or (2) calculated from the cumulative two-direction loads by applying factors for directional distribution and lane distribution. The 1993 AASHTO Guide offers the following basic equation:

Vehicle Speed
Although current design practices do not necessarily account for vehicle speed, it does influence pavement loading. In general, slower speeds and stop conditions allow a particular load to be applied to a given pavement area for a longer period of time resulting in greater damage. For HMA pavements this behavior is sometimes evident at bus stops (where heavy buses stop and sit while loading/unloading passengers) and intersection approaches (where traffic stops and waits to pass through the intersection) when mix design or structural design have been inadequate. In HMA pavement design, Superpave accounts for vehicle speed indirectly by applying a design pavement temperature adjustment for slow-moving or stopped vehicles.
The Equivalent Single Axle Load
Although it is not too difficult to determine a wheel or an axle load for an individual vehicle, it becomes quite complicated to determine the number and types of wheel/axle loads that a particular pavement will be subject to over its design life. Furthermore, it is not the wheel load but rather the damage to the pavement caused by the wheel load that is of primary concern. The most common historical approach is to convert damage from wheel loads of various magnitudes and repetitions (“mixed traffic”) to damage from an equivalent number of “standard” or “equivalent” loads. The most commonly used equivalent load in the U.S. is the 18,000 lb (80 kN) equivalent single axle load (normally designated ESAL). At the time of its development (early 1960s at the AASHO Road Test) it was much easier to use a single number to represent all traffic loading in the somewhat complicated empirical equations used for predicting pavement life.
Load Equivalency
Using the ESAL method, damage from all loads (including multi-axle loads) are converted to damage from an equivalent number of 18,000 lb. single axle loads, which is then used for design. A “load equivalency factor” represents the equivalent number of ESALs for the given weight-axle combination. The equation used to determine load equivalency can be quite complicated. As a rule-of-thumb, the load equivalency of a particular load (and also the pavement damage imparted by a particular load) is roughly related to the load by a power of four (for reasonably strong pavement surfaces). For example, a 36,000 lb. single axle load will cause about 16 times the damage as an 18,000 lb. single axle load.
Table 1 shows some typical load equivalencies (note that spreading a load out over two closely spaced axles reduces the number of ESALs). Figure 8, using some approximations, shows some general vehicle load equivalencies – note that buses tend
| Load | Number of ESALs |
|---|---|
| 18,000 lb. single axle | 1.000 |
| 2,000 lb. single axle | 0.0003 |
| 30,000 lb. single axle | 7.9 |
| 18,000 lb. tandem axle | 0.109 |
| 40,000 lb. tandem axle | 2.06 |
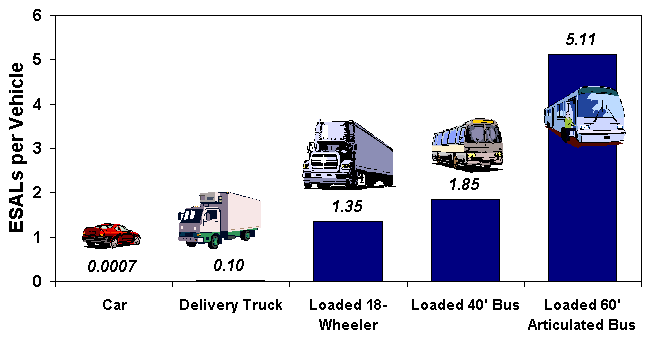
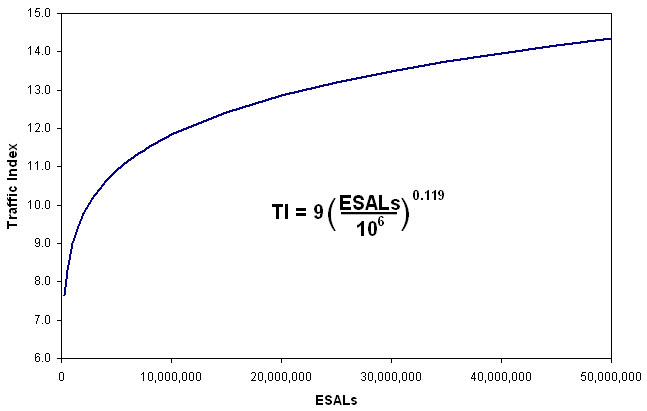
- Traffic Index (TI). The traffic index is associated with the California method of pavement structural design. Essentially, it has evolved in to a way of expressing ESALs as a single number or index (see Figure 9).
Load Spectra
The 2002 Guide for the Design of New and Rehabilitated Pavement Structures (NCHRP 1-37A) has gone away from the ESAL approach and adopted a load spectra approach. In essence, the load spectra approach uses the same traffic data that the ESAL approach uses only it does not convert the loads into ESALs – it maintains the data by axle configuration and weight. This information can then be used with a series of mechanistic-empirical equations to develop a pavement structural design. Some key advantages of the load spectra approach are:
-
-
- It is compatible with the FHWA’s Traffic Monitoring Guide (TMG) and thus many agencies are already collecting the appropriate data.
- It offers a hierarchical approach to traffic data input depending upon the users needs and resources. There are three levels of potential input:
- Level 1 Inputs – Use of volume/classification and axle load spectra data directly related to the project.
- Level 2 Inputs – Use of regional axle load spectra data and project-related volume/classification data.
- Level 3 Inputs – Use of regional or default classification and axle load spectra data.
- It already includes information on traffic distribution including directional, lane and temporal distribution (if needed) as well as traffic growth rates.
-
- Evaluation of Present Legislation and Regulations on Tire Sizes, Configurations and Load Limits. Research Report WA-RD 59.1, Washington State Department of Transportation. Olympia, WA.↵


